Educators might be surprised that I don’t teach factoring as a part of solving quadratic equations. That is, I don’t teach in a quadratics units how to recognize \(x^{2}-5x+6\) as \((x-2)(x-3)\) and \(6x^{2}-7x-5\) as \((2x+1)(3x-5)\), for example.

I omit factoring from the quadratics unit, and do it later on in the year, for one strong reason.

Factoring yields expressions that are associated with pictures of rectangles. But solving quadratics is a story of SYMMETRY, using the symmetry of a square to our advantage. (And making full use of symmetry is a powerful technique in mathematics.) Let’s not muddy the story by bringing in a non-symmetrical idea out-of-the-blue.

The technique we exploit in solving quadratics comes from noticing that we can easily solve a square problem: \(a^2=9\). We cannot, however, solve a rectangle problem  \(a\times b = 9\) without extra information. (There are infinitely many solutions to \(ab = 9\).) The symmetry of the square is a potent tool to use.

WHEN TO TEACH FACTORING

I’d prefer the answer to be never, except when it is truly needed in a study of Galois Theory.

My concern with the introduction of factoring in a high-school curriculum is that it chiefly introduced as a technique to answer questions that have been designed to be solved by factoring. Most quadratics never factor nicely. (Care to guess the factors of \(x^{2}+x+1\)?).

Because it is a bit of a false construct in the high-school curriculum, I would prefer to bump the study of factoring to a unit on graphing polynomials. (At least this offers a modicum of motivation about why one might care to factor: One can nut one’s way through thinking what the graph of \(p\left(x\right)=\left(x-5\right)^{3}\left(x-1\right)\left(x+2\right)^{51}\left(x+4\right)^{300}\left(x+10\right)\) looks like.)

Of course, moments of needing to factor might occur earlier and I have no trouble with asking students to treat factoring as a logic puzzle about the area model:

Can you find “nice” numbers that fit this rectangle?

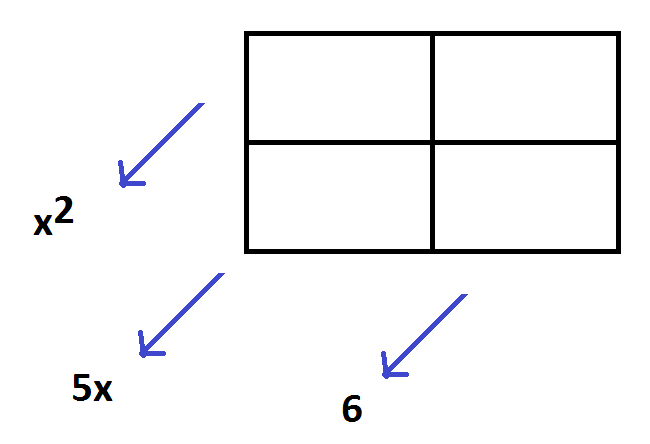
But keep this work as a separate exercise from a first introduction to solving quadratics. The story of the symmetry of a square needs to well and truly shine through and settle in. Keep factoring very separate, at least at first.

Please join the conversation on Facebook and Twitter and kindly share this page using the buttons below.

Facebook

Twitter

Resources