Here’s an unusual way to divide by nine.

To compute \(21203 \div 9\), say, read “\(21203\)” from left to right computing the partial sums of the digits along the way

and then read off the answer

\(21203\div 9 = 2355 \; R8\).

In the same way,

\(1033 \div 9 = 114 \; R7\)

and

\(2222 \div 9 = 246 \; R8\).

Can you explain why this trick works?

Here’s the approach I might take: For the first example, draw a picture of \(21203\) in a \(1 \leftarrow 10\) machine, but think of nine as \(10-1\). That is, look for copies of

in the picture.

Use an \(1 \leftarrow x\) machine to compute each of the following

a) \(\dfrac{x^{2}-1}{x-1}\)         b)  \(\dfrac{x^{3}-1}{x-1}\)      c) \(\dfrac{x^{6}-1}{x-1}\)      d) \(\dfrac{x^{10}-1}{x-1}\)

Can you now see that \(\dfrac{x^{number}-1}{x-1}\) will always have a nice answer without a remainder?

Another way of saying this is that \(x^{number}-1 = \left(x-1\right) \times \left(something\right)\).

For example, you might have seen from part c) that \(x^{6}-1 = \left(x-1\right)\left(x^{5}+x^{4}+x^{3}+x^2+x+1\right)\). This means we can say, for example, that \(17^{6}-1\) is sure to be a multiple of \(16\)! How? Just choose \(x=17\) in this formula to get

\(17^{6}-1 = \left(17-1\right) \times \left(something\right)=16\times \left(something\right) \).

a) Explain why \(999^{100}-1\) must be a multiple of \(998\).

b) Can you explain why \(2^{100}-1\) must be a multiple of 3, and a multiple of 15, and a multiple of 31 and a multiple of 1023? (Hint: \(2^{100}=\left(2^2\right)^{50}=4^{50}\), and so on.)

c) Is \(x^{number}-1\)  always a multiple of \(x+1\)? Sometimes, at least?

d) The number \(2^{100}+1\) is not prime. It is a multiple of \(17\). Can you see how to prove this?

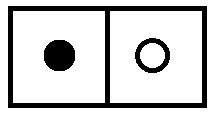
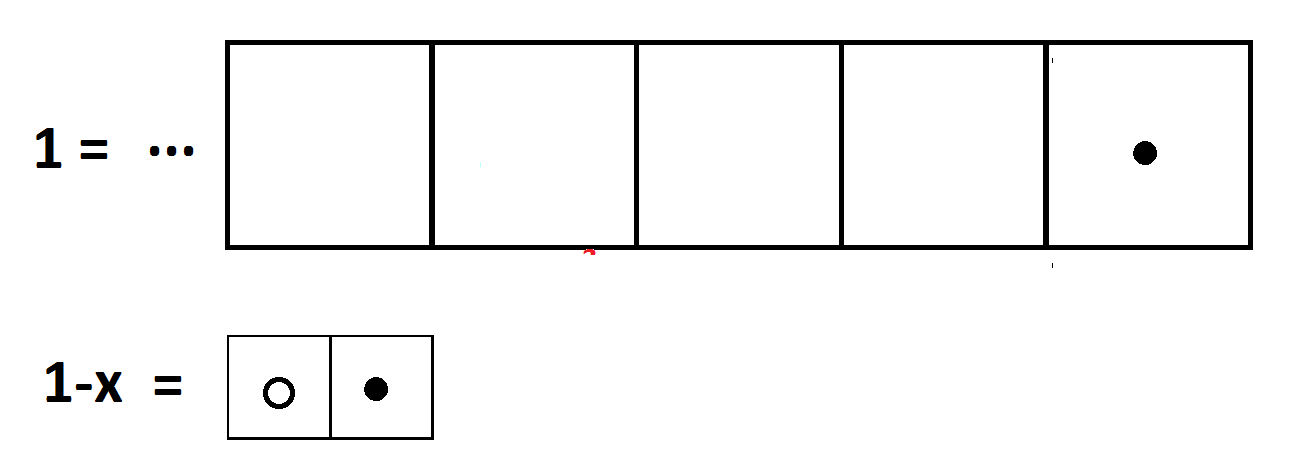
 Here is a picture of the very simple polynomial \(1\) and the polynomial \(1-x\).

Can you compute \(\dfrac{1}{1-x}\)? Can you interpret the answer?

(We’ll explore this example in the next chapter.)

Math enthusiast Baron Hansz has described an terrific way to perform long multiplication in any base you like — even fractional ones — with absolute ease! He brilliantly combines the area model with Exploding Dots thinking. This video bounces of his video, giving my take on explaining Baron’s lovely approach.

Her’s my take on explaining Baron’s lovely approach.

Click here for VIDEO

And here’s Baron’s video with the fellow himself explaining his method.

Click here for VIDEO

What do you think of this approach?