4.5 A Historic Problem

The Astounding Power of Area

4.5 A Historic Problem

Course Home

The start of probability theory can essentially be pinpointed to a single moment in time. In 1654 French nobleman Chevalier de Méré wrote to prominent mathematician Blaise Pascal asking for advice on the general “problem of points” (among other issues in making bets in gambling). These problems go as follows

Two friends each lay down $100 in a friendly “best of seven” tennis game, say. But rain interrupts play after just four games with one person having won three games and the other just one. How should the $200 be divvied between the two players so as to properly reflect the likelihood of each winning?

Pascal shared his thoughts with Pierre de Fermat. It is with de Méré’s inquiry that probability theory was born as a subject to be developed and deeply studied on its own right.

Comment: Italian mathematician Girolamo Cardano (1501-1576) actually worked with ideas akin to classical probability theory before this but did not publish his work. And of course gambling games have been in existence for centuries and scholars have wondered about, analyzed, and computed likelihoods of certain results. But the first definitive analysis of “chance” began with the work of Pascal and Fermat.

I invite you to analyze de Méré’s problem. How would you approach the problem described above? What beginning assumptions would you make? What solution would you personally offer for splitting the pot between the two players?

Don’t read on until you have thought through this problem yourself.

SOME POSSIBLE SOLUTIONS

Here are four possible solutions to the challenge. Start by giving the two gentleman names, A (for Albert) and B (for Bilbert), say, and suppose A has won three games and B just one.

Possible Solution 1: Just give each player back his $100 and play another day.

Possible Objection: A will likely complain that he was on the verge of winning and should receive more than this amount.

Possible Solution 2: Give player A $150 and player B $50 to reflect the ratio of their wins thus far.

Possible Objection: A might argue that if he were to win the next game he’d be given the full $200 and player B $0. This is better than the $160 and $40 distribution the 4:1 win ratio would suggest. Ratios undercut what A deserves.

Possible Solution 3: Let’s use garden paths.

Suppose A and B are equally strong players, each just as likely to win any particular game. Here is the garden path analysis under this assumption.

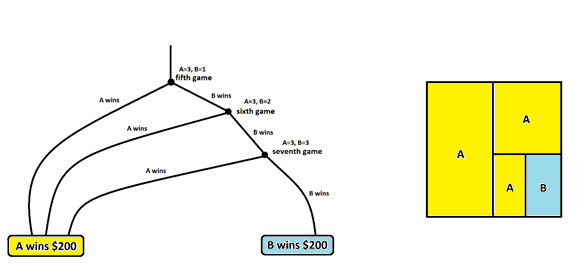
We see in this scenario that B has a one-eighth chance of winning the pot. This suggests splitting the pot in a 7:1 ratio, which corresponds to giving A $175 and B $25.

Possible Objection: A would argue that the evidence from the first four games played (three wins for A, one for B) suggests that he beats player B 75% of the time, not just 50% of the time.

Possible Solution 4: Let’s modify the garden path model to follow the assumption that A has a 75% chance of winning any particular game.

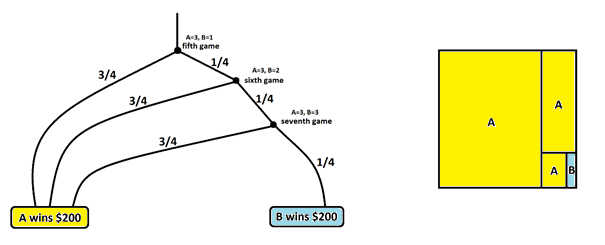
We see in this scenario that B has a one in sixty-four chance of winning the pot. This suggests the split $196.875 for A and $3.125 for B. (How should they deal with the half penny?)

Possible Objection: B claims that he was “laying low” for the first few games for a psychological advantage and that he actually wins 95% of all games played!

Challenge: Find yet another reasonable solution to de Méré’s challenge.