A DIFFERENT BASE TWO

a) Verify that a \( 2 \leftarrow 4\) machine is a base-two machine. (That is, explain why \(x=2\) is the appropriate value for \(x\) in the picture below.)

b) Write the numbers 1 through 30 as given by a \( 2 \leftarrow 4\) machine and as given by a \( 1 \leftarrow 2\) machine.

c) Does there seem to be an easy way to convert from one representation of a number to the other?

(Care to explore representations in \( 3 \leftarrow 6\) and \( 5 \leftarrow 10\) machines too?)

d) How does long division work in this machine? Can you compute \(30 \div 5\) in it? Can you compute \(1 \div 3\)?

YET ANOTHER BASE TWO

Now consider a \( 1|1 \leftarrow 3\) machine. Here three dots in a box are replaced by two dots: one in the original box and one one place to the left. (Weird!)

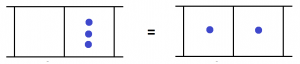
a) Verify that a \( 1|1 \leftarrow 3\) machine is also a base two machine.

b) Write the numbers 1 through 30 as given by a \( 1|1 \leftarrow 3\) machine. Is there an easy way to convert the \( 1|1 \leftarrow 3\) representation of a number to its \( 1 \leftarrow 2\) representation, and vice versa?

c) How does long division work in this machine? Can you compute \(30 \div 5\) in it? Can you compute \(1 \div 3\)?

A DIFFERENT BASE THREE

 Here’s a new type of base machine. It is called a \( 1|-1 \leftarrow 0|2\) machine and operates by converting any two dots in one box into an antidot in that box and a proper dot one place to the left. It also converts two antidots in one box to an antidot/dot pair.

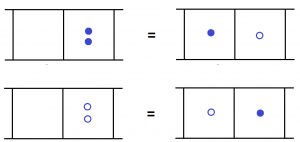
a) Show that the number twenty has representation \(1|-1|1|-1\) in this machine.

b) What number has representation \(1|1|0|-1\) in this machine?

c) This machine is a base machine:

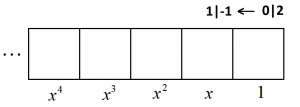
Explain why \(x\) equals 3.

Thus the \( 1|-1 \leftarrow 0|2\) machine shows that every number can be written as a combination of powers of three using the coefficients \(1\), \(0\), and \(-1\).

d) A woman has a simple balance scale and five stones of weights 1, 3, 9, 27, and 81 pounds.

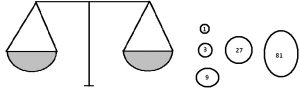
I place a rock of weight 20 pounds on one side of the scale. Explain how the women can place some, or all, of her stones on the scale so as to make it balance.

e) Suppose instead I place a 67 pound rock on the woman’s scale. Can she make that stone balance too?

Please join the conversation on Facebook and Twitter and kindly share this page using the buttons below.

Facebook

Twitter

Resources