Okay. Now that we are feeling really good about doing advanced algebra, I have a confession to make. I’ve been fooling you!

I’ve been choosing examples that are designed to be nice and to work out just beautifully. The truth is, this fabulous method of ours doesn’t usually work so nicely.

Consider, for example, \(\dfrac{x^3-3x+2}{x+2}\).

Do you see what I’ve been avoiding all this time? Yep. Negative numbers.

Here’s what I see in an \(1 \leftarrow x\) machine.

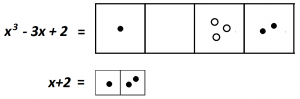
We are looking for one dot next to two dots in the picture of \(x^{3}-3x+2\).  And I don’t see any!

So what can we do, besides weep a little? Do you have any ideas?

It is tempting to say that we should just unexplode some dots. That’s a brilliant idea! Except … we don’t know a value for \(x\) and so don’t know how many dots to draw when we unexplode. Bother!

We need some amazing flash of insight for something clever to do. Or maybe polynomial problems with negative numbers just can’t be solved with this dots and boxes method.

What do you think? Any flashes of insight?

Please join the conversation on Facebook and Twitter and kindly share this page using the buttons below.

Facebook

Twitter

Resources